Marginal Rate of Substitution (MRS)

def. Marginal Rate of Substitution. $MR{S}_{1,2}$ asks “How much of $x_2$ can you give me for one unit of $x_1$?”

• ${\text{MRS}}_{1,2}$ means MRS of good $x_1$ for good $x_2$, i.e. “one unit of $x_1$ for how many units of $x_2$?""

$${\text{MRS}}_{1,2}:=-\frac{d{x}_2}{d{x}_1}=-\frac{\frac{\partial u}{\partial x_1}}{\frac{\partial u}{\partial x_2}}$$

x_{1},x_{2} changes spots in the nominator/denominator in the final formula.

• The tangent of Utility Function at a certain point is the MRS at that specific point (more accurately, its absolute value of the tangent)
• Convexity Assumption causes MRS to decrease (=Diminishing MRS) rightward (the more of good $x_1$ we consume, the more we would like $x^2$).
• When two people have different MRS, then they can trade for both of their utility.
  • e.g. $MR{S}_A=-3$, $MR{S}_B=-\frac{1}{2}$
  • ⇒ they can trade at any rate where $MR{S}_A<\text{rate}<MR{S}_B$ to both of their benefit.

Deriving the MRS Formula §

We’re looking for a place on the scalar field provided by $u(x_1,x_2)$ where the gradient is zero. ⇒ Thus we need to find a point where the following is true:

$$\nabla u(x_1,x_2)=\frac{\delta u}{\delta x_1}d{x}_1+\frac{\delta u}{\delta x_2}d{x}_2=0$$

By the definition of MRS it’s the gradient of a indifference curve, i.e. the gradient of a level curve in the field:

$$MRS=-\frac{d{x}_2}{d{x}_1}=-\frac{\partial u/\partial x_1}{\partial u/\partial x_2}$$

Changes in MRS §

• MRS can change due to exogenous factors; in this case the whole indifference curve will change accordingly too.
• e.g. After 9.11., air travel is perceived by consumers to be less safe.

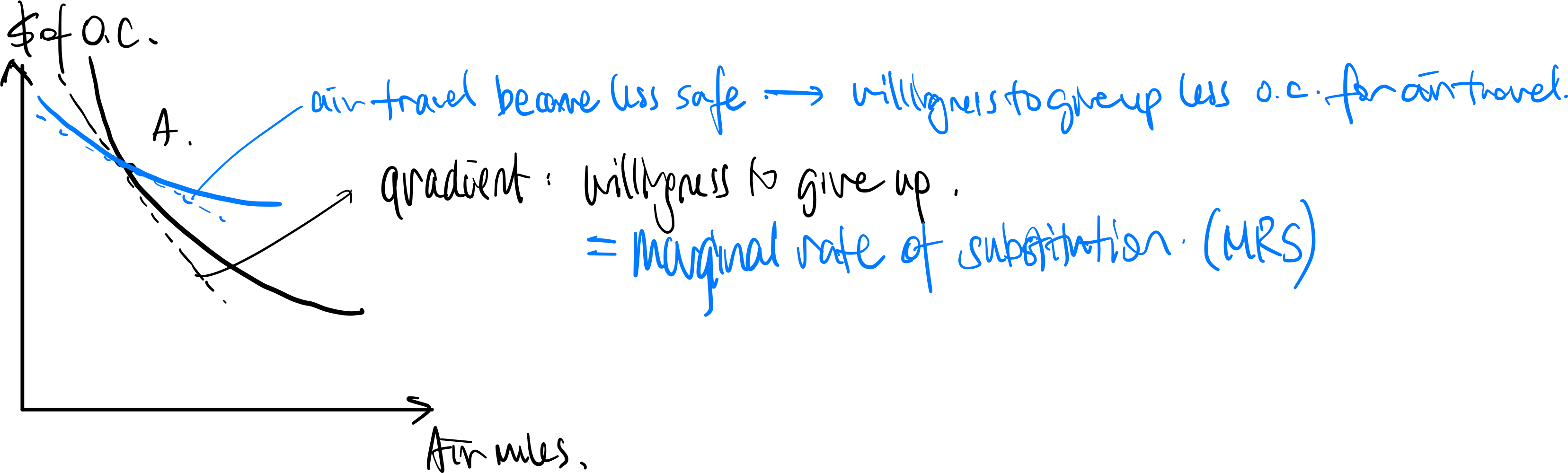
• Observe that air travel becoming less safe, causes the MRS to decrease, as one is willing to trade less money for flying by air.
• You can think of this from a different perspective by instead of thinking of air safety as an exogenous factor, actually quantifying air safety as a measure as well, and graphing it together.
• Graphing air safety as the third axis shows that we can in fact visualize the decrease in MRS of air miles and $‘s of other consumption.